This report represents the second in a series of planned flight tests for the purpose of determining performance and operating parameters of the Kopp manufactured BD-4 experimental (homebuilt) airplane. Flight Test 1 was conducted for determination of the drag polar and power required for level flight. Data was collected at various weights and altitudes and then standardized to sea level, max gross weight conditions during data reduction. This key information forms the foundation by which operational flight performance parameters are determined. A summary of the results obtained from Flight Test 1 are shown in the table below.

Table 1 Summary Table for Kopp BD-4

Altitude / Weight	Max C_l/C_d	Min Thrust Required
3000 ft / 1950 lbs	8.8235	217.87 lbs
7500 ft / 2130 lbs	9.0329	229.63 lbs
Parameters	Drag Polar	Power Curve
C_do	0.0440	0.0425
e	0.7031	0.6507
Altitude	Minimum Thrust Horsepower Required
3500 ft 1950 lbs	52.33 HP
7500 ft 2130 lbs	60.59 HP
Standardized	59.16 HP
Kopp BD-4 Summary Table Test conducted 27 July, 2000 Data to be added upon further testing

Performance parameters calculated in table one are a result of airframe configuration only and are independent of installed propulsion. This flight test introduces propulsion system effects for determination of relationships between the two independent data sets. At the conclusion of this report table 1 is expanded to include: maximum rate-of-climb (R/C_max), V_x (max angle-of-climb airspeed), V_y (R/C_max airspeed) and max angle of climb (AOC).

Excess power, defined as the difference between power available and power required for level unaccelerated flight results in either a climb or an acceleration. Power available is determined by the installed propulsion system. Two data collection methods are employed for excess power determination, these are; level acceleration and sawtooth climb methods. Data collected is reduced to determine climb rate, excess power and in conjunction with power-required data, power available. The power available calculated from test data is compared to values determined using engine power charts and propeller performance mapping software supplied by Hartzell Propeller Inc. as a means to validate (or invalidate) the flight test method and the supplied propeller and engine data. Comparison between flight test methods is also included.

Three different altitudes, 1500ft, 3000ft and 7500ft were flown during the level acceleration test and a single 3000ft flight was flown using the sawtooth climb method

The Kopp BD-4’s designed mission is that of medium range cross-country cruiser and general recreational aircraft. The main focus of these flight tests is to determine how its performance aids or deters fulfillment of its designed mission.

Kopp BD-4

The Kopp BD-4 is a single engine, 4 place IFR capable homebuilt airplane. It is equipped with a Lycoming O-360-A1A 180 HP horizontally opposed, direct drive, normally aspirated engine turning a 74” Hartzell 7666-2 constant speed propeller.

Wings

The BD-4 has a cantilever high wing with a 64-415 modified airfoil. A plain flap of 71% span and cord of 15% MAC can deflect from 0°-30°. Ailerons of the sealed configuration, also have a chord of 15% MAC and are deflected differentially by 1” diameter torque tubes. The unique tubular spar and metal-to-metal bonding used in the wings kept costs of construction and maintenance low, weight light and construction simple. Three components comprise the entire cantilever spar design; the center section and two slightly larger wing tubes which are all bolted together with four AN4 bolts ( not so jokingly referred to as Jesus bolts).

Fuselage

The all-metal fuselage was fabricated entirely of simple flat aluminum gussets and varying length angles of different dimension. The entire assembly is bolted together “erector set style” using the highest quality AN hardware. .020” 2024 T3 aluminum skin is bonded and blind riveted to the structure and together form a sturdy, dependable airframe rated to a limit load of +-6 g’s.

Empennage

The horizontal tail is of the “all-flying” variety found on many Piper airplanes. The stabilator consists of a single tubular spar and several rib sections formed into a 63-009 airfoil. The vertical tail is of similar construction.

Table 2 below is a detailed listing of all Kopp BD-4 specifications..

Table 2Kopp BD-4 Specifications

Wing Span	25.6 ft	Cabin Width	42”
Wing Chord	4 ft	Cabin length	89”
Wing Area	102.33 ft²	Cabin height	41”
Aspect Ratio	6.4	Fuel Capacity	60 gal
Aileron Area	3.5 ft²	Elevator Def up	15°
Flap Area	8 ft²	Elevator Def down	6°
Flap Span	71%	Trim Tab Up	18°
Aileron Defl Up	25°	Trim Tab Down	10°
Aileron Defl Down	17°	Rudder Deflection	+- 25°
Length	21.4 ft	Flap Deflection	0°-30°
Horizontal Stab Span	7.3 ft	Max Gross Weight	2200
Horizontal Chord	3 ft	Empty Weight	1412 lbs
Horizontal Stab Area	21.9 ft²	Useful Load	788 lbs
Horizontal Stab AR	2.4	Wing Loading	21.5 lbs/ft²
Vertical Stab Area	12 ft²	Power Loading	12 lbs/BHP

Part I - Instrument Position Errors

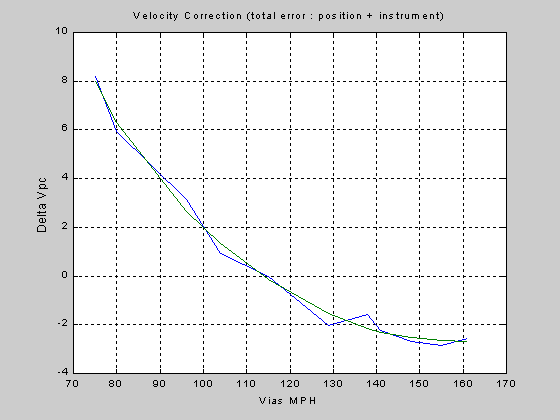
Pitot static position errors were determined and reported in flight test 1. Figure 1 below is a plot of ΔV_pc vs. V_ias(indicated) where ΔV_pc represents the velocity correction which when applied to V_ias results in V_cas (calibrated airspeed). V_cas is then adjusted for test day density altitude to arrive at V_tas(true airspeed).

Figure 1 Airspeed Position Error Plot

mph

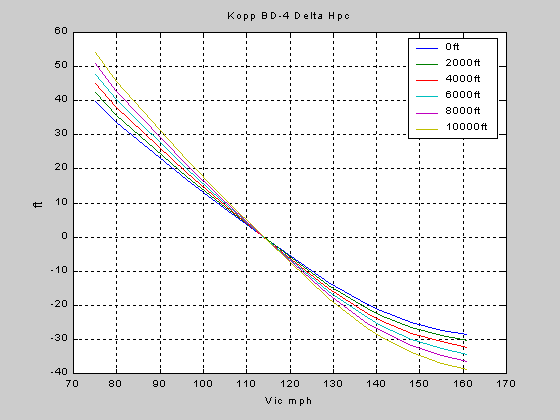
These corrections are important since the primary measurement sources during flight are the installed aircraft pitot-static instruments (airspeed, altimeter) and without valid corrections, results would be erroneous. In addition to the airspeed correction the altimeter must also be corrected according to the following relationship:

, where ΔH_pc is the correction applied to indicated altitude as follows:

H_{i (indicated)} + ΔH_pc= H_c _{(true altitude)}

As can be seen by the equation above, DH_pc is dependent upon altitude, requiring a correction to be applied to each recorded altitude throughout the airspeed range flown, as indicated in the figure below.

Figure 2 Hpc Plot

Part II – Flight Test

General Information

This test was conducted in the Kopp BD-4 on 10 Aug, 2000 departing from Monterey Peninsula Airport (MRY) at 10:00 am. Conditions at take-off were:

Wind: 290/8

Alt: 30.04

Sky Clear

Rwy: 28R

Crew and altitude assignments were as follows:

Table 3 Crew and altitude assignments

Crew - level acceleration runs	Altitude	Gross Weight (approx)
LT Ken Kopp / LT Anthony Fortesque	3000 ft	2175 lbs
LT Ken Kopp / LT Anthony Fortesque	7500 ft	2130 lbs
Crew – Sawtooth Climb	Altitude	Gross Weight (approx)
LT Ken Kopp / Maj. Jim Hawkins	3000 ft	2025 lbs

The test area was restricted to Salinas Valley from Salinas to 15 miles South East of King City. Crew coordination and a thorough test procedures briefing preceded each flight. Data collection sheets were developed, printed and discussed in detail prior to flight as well. Specific responsibilities were delegated as follows:

Table 4 Flight Responsibilities

Responsibility	Pilot at the Controls	Pilot Not at the Controls
Flight Safety	Primary	Secondary
Airwork	Primary
Test Procedure		Primary
Data Recording		Primary
Communications	Primary	Secondary
Navigation	Secondary	Primary
Visual Lookout	Secondary	Primary
Emergencies	Primary	Secondary

ATC flight following was utilized to the maximum extent possible to aid in collision

avoidance. King City and Salinas Muni were designated primary diverts in the event an emergency due to mechanical failure or weather occurred.

To minimize parallax error the left seat pilot remained at the controls while the right seat pilot recorded data.

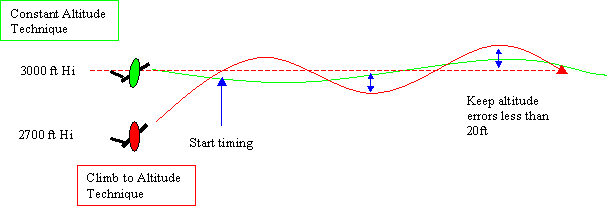
Level Acceleration Method

Climb to Altitude Technique

In this method the aircraft is slowed to V_mca (minimum controllable airspeed) 2-3 hundred feet below the target altitude. Full power is smoothly applied and the aircraft allowed to climb. As the aircraft approaches target altitude a pitch correction is applied to level the aircraft on target altitude while accelerating to V_max. Upon reaching altitude a timer is started and elapsed time recorded at predetermined airspeed intervals. Pilot technique, solid crew coordination and practice are required to obtain any degree of accuracy in this method. Indicated altitude should be maintained as precisely as possible and any indicated rates of climb or descent should be noted if possible. Data recorded is Hi, Vias, elapsed time, MP, RPM and OAT.

Constant Altitude Technique

This technique requires the aircraft to be slowed to V_mca at the specified target altitude and to smoothly apply power while recording elapsed time between specified airspeed intervals during the ensuing acceleration. This technique is extremely difficult in the Kopp BD-4 due to torque effects on yaw and roll making altitude control difficult during the first few seconds of data collection, therefore; the climb to altitude technique is preferred.

Figure 2 Level Acceleration Techniques

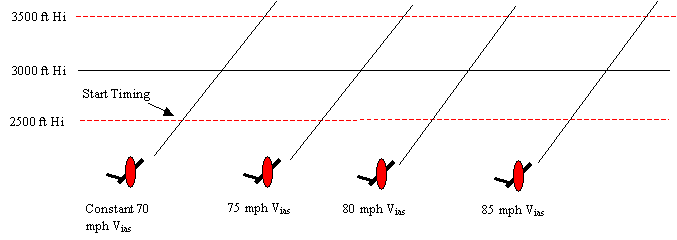
Sawtooth Climb Method

In this method a test band of ± 500 feet is set around a target altitude (3000ft in this case) while the aircraft is slowed to V_mca1-2 hundred feet below the lower test band altitude. While maintaining constant V_ias , full power is applied and a climb commenced. Upon reaching the lower limit a timer is started and elapsed time between hundred foot intervals is recorded, along with V_ias,MP, RPM and OAT. This cycle is repeated at 5 mph airspeed intervals throughout the range of interest.

Figure 3 Sawtooth Climb Method

Part III Data Reduction

Level Acceleration Data (climb to altitude technique)

Measurements were recorded and reduced for indicated altitudes of 1500, 3000 and 7500 ft. Five runs were conducted for each altitude to facilitate averaging for the purpose of minimizing deviations during each run. A running fuel burn was tallied to account for change in gross weight over the test period. For purposes of brevity, only data for the 3000 feet case will be discussed in the body of the text, however; plots of all pertinent data will be displayed and discussed. The remaining data is located in the appendix.

Raw data collected during the 3000 ft run is displayed in the table below.

Table 5 Level Acceleration 3000 feet

Data Sheet		3000 Ft
Pwr Avail
start fuel	G W	Wind	Alt	Temp	Srt T	T/O T
60	2195	350/6	30.06	17	11:12	11:23
Climb pwr	lvl T	trans pwr	Climb BR	Run BR	Transit BR	Descent BR
27/27	11:27	27/20	13	13	7.8	5.0

PA	OAT	MP	RPM	Start GW	End GW	Ave GW
3000	66	26.2”	2700	2182.035	2165.0	2173.5
Clock TIme	11:35	11:40	11:46	11:50	11:54
IAS	Time	Time	Time	Time	Time	Ave Time
70	0	0	0	0	0	0.0
75		3	4	2	2	2.8
80	3	5	5	5	3	4.2
85		7	6	8	5	6.5
90	5	8	9	10	8	8.0
95		10	10	11	9	10.0
100	10	15	12	12	10	11.8
105	15	19	16	14	13	15.4
110	18	23	20	17	15	18.6
115	24	25	23	19	18	21.8
120	27	28	28	22	23	25.6
125	31	35	35	28	29	31.6
130	35	40	40	32	35	36.4
135	43	44	44	38	40	41.8
140	52	50	53	45	48	49.6
145	59	59	64	54	59	59.0
150	77	97	83	81	79	83.4

The first step in data reduction is to apply static position corrections to both airspeed and altitude as mentioned in part 1. The result of these corrections is displayed in the table below. Additionally, values for standard temperature, pressure and density for each true altitude (H_c) are computed via atmospheric table interpolation.

Table 6 DV_pc & DH_pc corrections 3000ft

3000ft	rho std		sigstd	gama	ao	Ti
	0.0022		0.917722	1.4	1116.29	66
Vias	Delt Vpc	Vcas	Hi	delt Hpc	Hc	Ts	Ps	rho	rho std
mph	mph	mph	ft	ft	ft	F	lb/ft^2	slug/ft^3	slug/ft^3
70	10.09	80.09	3000.00	51.54	3051.54	46.94	1893.29	0.002096268	0.002172
75	8.03	83.03	3000.00	43.93	3043.93	46.96	1893.83	0.002096858	0.002173
80	6.36	86.36	3000.00	37.13	3037.13	46.99	1894.30	0.002097385	0.002173
86	4.75	90.75	3000.00	29.82	3029.82	47.01	1894.81	0.002097952	0.002173
90	3.85	93.85	3000.00	25.31	3025.31	47.03	1895.13	0.002098301	0.002174
95	2.88	97.88	3000.00	19.96	3019.96	47.05	1895.50	0.002098716	0.002174
100	2.03	102.03	3000.00	14.79	3014.79	47.07	1895.86	0.002099116	0.002174
105	1.27	106.27	3000.00	9.72	3009.72	47.09	1896.22	0.002099509	0.002175
110	0.58	110.58	3000.00	4.69	3004.69	47.10	1896.57	0.002099899	0.002175
115	-0.03	114.97	3000.00	-0.29	2999.71	47.12	1896.92	0.002100285	0.002175
120	-0.59	119.41	3000.00	-5.17	2994.83	47.14	1897.26	0.002100663	0.002176
125	-1.08	123.92	3000.00	-9.86	2990.14	47.16	1897.59	0.002101026	0.002176
130	-1.50	128.50	3000.00	-14.24	2985.76	47.17	1897.90	0.002101366	0.002176
135	-1.84	133.16	3000.00	-18.18	2981.82	47.18	1898.17	0.002101671	0.002177
140	-2.10	137.90	3000.00	-21.55	2978.45	47.20	1898.41	0.002101933	0.002177
145	-2.29	142.71	3000.00	-24.28	2975.72	47.21	1898.60	0.002102144	0.002177
150	-2.40	147.60	3000.00	-26.34	2973.66	47.21	1898.74	0.002102304	0.002177
						Interpolation Values for 3000 ft
						A	Ts	Ps	rho std
						2500	509.77	1931.9	0.0022079
						3500	506.21	1861.9	0.0021429

Rate of climb, a measure of excess power, is calculated according to the equation below:

_,where V_tas is true airspeed in ft/sec and dh/dt is the change in altitude with respect to time or R/C in ft/sec. dV_tas/dt is the acceleration.

With static position corrections applied determination of V_tas for each data point is accomplished by solving the equation below.

, where σ is the ratio of air density at each H_c to standard sea level density. The slope of a tangent line to the curve resulting from a plot of V_tas vs. time is equal to the instantaneous acceleration at that point or dV_tas/dtas shown in the figure below.

Figure 4 Velocity vs. Time

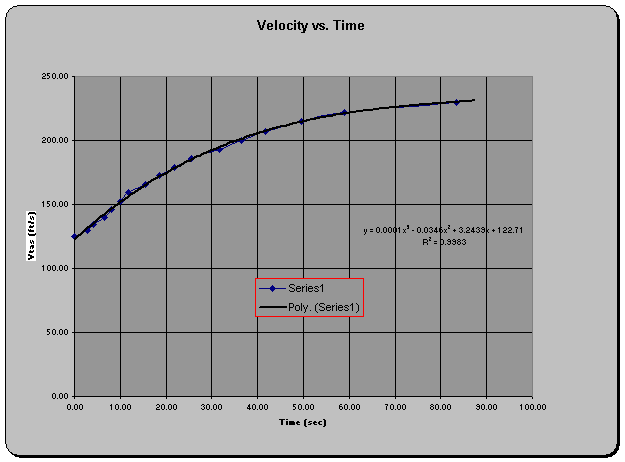
To determine values of dV_tas/dt analytically, a third order curve fit is applied to the data plotted above. The resulting equation is then differentiated with respect to time to generate an equation for dV_tas/dt as shown below:

differentiating with respect to time results in:

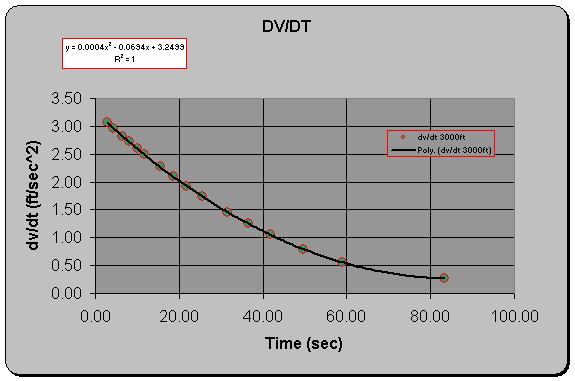
Applying the recorded times during the flight to the above equation results in values of acceleration for each data point as shown in the figure below.

Figure 5 Dv/Dt

Determination of uncorrected R/C for test day conditions and gross weight requires only that the equation be solved for each data point. The result of this calculation is displayed in the figure below.

Figure 6 R/C uncorrected test day 3000ft

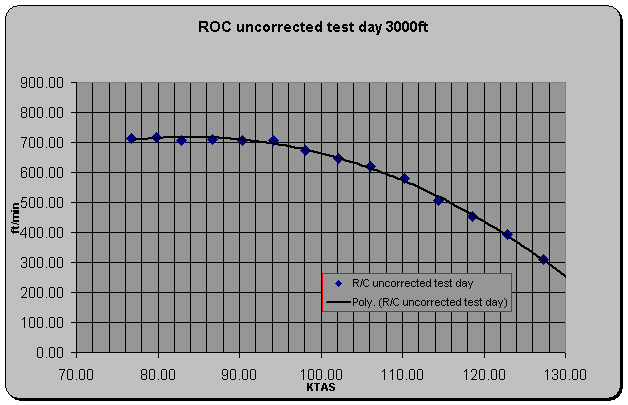
The R/C depicted above is termed uncorrected because data leading to calculated values of acceleration is corrupted by altitude management and static position errors during the run. A quick glance back at table 6 and figure 2 shows that although indicated altitude H_i remained constant the aircraft is actually descending as it accelerates.

In fact, 77.8 feet was lost during the run. A plot of Hc vs. Time for the run is shown in the figure below.

Figure 7 Delta Hc vs TIme

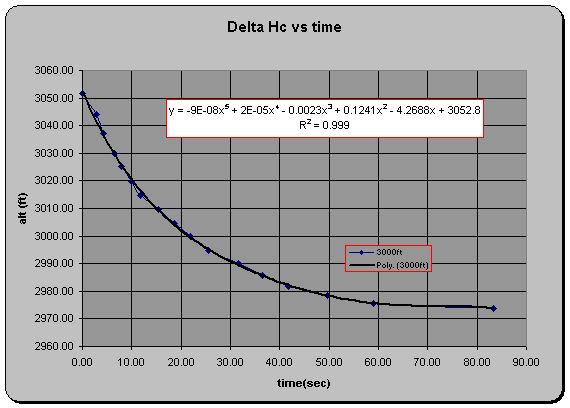
This actual R/D (rate of descent) causes the aircraft to accelerate more quickly resulting in an optimistically higher value of calculated dh/dt. Fortunately, this effect is easily corrected by simply subtracting the actual R/D directly from calculated values of dh/dt to arrive at the airplanes actual R/C climb under test day weight and atmospheric conditions. Several methods can be used to estimate the R/D during the run. A curve fit of the plot above can be differentiated to arrive at an equation for dh/dt_local . Unfortunately as the order of the fit increases so to does the sensitivity of its derivative. For this reason, the local R/D at each point was calculated by the following relationship:

. Which is simply the average slope between each two test points. A plot of the determined descent rate is shown below.

Figure 8 Calculated Descent Rate Correction

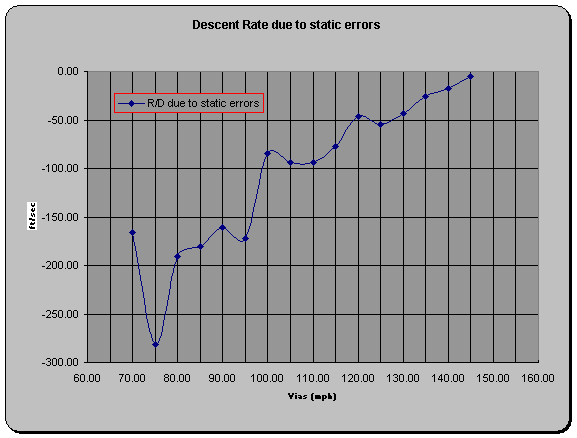
Test day R/C is then determined by:

Test day R/C is useful for validation of known data points or for comparison with similar test day condition results, but to enable prediction of aircraft performance during other than test day conditions , several standardizing corrections must be applied to the calculated R/C_{test day}. Otherwise, test data would be required for each weight, altitude and temperature combination, which is unrealistic. The additional corrections are listed below in the order they are to be applied.

Power correction (DR/C_power) – applied to account for change in power generated due to non-standard temperature.
Inertial correction (DR/C_inertial) – applied to account for non-standard weight
Induced drag correction (DR/C_ind) – applied to account for non-standard weight.

Knowing full power was used for all climb performance calculations, the power correction is determined by:

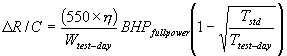, where T_std can be any temperature of interest, not necessarily standard atmospheric temperature.

and the inertial correction by:

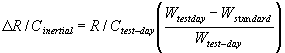, where W_standard is any weight of interest.

Because an airplane flying at higher gross weight must fly a higher angle of attack for a given airspeed the induced drag of the heavier plane will necessarily be higher. Recalling that induced drag is a function of C_L² and C_Lis a function of lift, in level unaccelerated flight lift equals the weight of the aircraft. Therefore, as weight increases so does the value of C_L² for a given airspeed and thus induced drag increases as well. This results in more power required to maintain level flight at the higher weight for that airspeed. An increase in power required results in decreased excess power, since installed power is independent of airframe aerodynamics and remains constant for a given airspeed, resulting in a decreased R/C. The induced drag correction is given by:

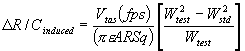, where q is the dynamic pressure at the test altitude and temperature. Combining test day R/C with each correction for specified W_std and T_std results in R/C corrected to those weights and temperatures and is given by:

Arbitrarily choosing values of W_std and T_stdto be 2200 lbs and standard atmospheric temperature at each H_c determined in table 6 and applying each correction to R/C_{test day}, results in values of R/C_std shown in the following table.

Table 7 3000ft R/C reduced data

		Ws (lbs)	e	AR	S (ft^2)
		2200	0.7031	6.4	102.33
	dh/dt	climb/desc	dh/dt	Power	inertial	ind drag	DH/DT
Dv/dt	uncorrected	correction	test day	correction	correction	correction	corrected
(fps)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)
3.06	713.23	-166.01	547.22	27.55	-7.00	-16.28	551.49
2.97	715.94	-281.29	434.64	28.22	-5.64	-15.65	441.58
2.82	706.97	-190.83	516.14	28.81	-6.63	-15.06	523.26
2.72	709.73	-180.12	529.60	29.10	-6.80	-14.40	537.51
2.60	708.18	-160.56	547.62	29.91	-7.03	-13.80	556.70
2.49	707.43	-172.22	535.21	30.46	-6.89	-13.24	545.54
2.28	674.93	-84.57	590.35	31.01	-7.57	-12.71	601.09
2.10	647.85	-94.28	553.58	31.47	-7.12	-12.21	565.71
1.93	619.46	-93.35	526.11	31.76	-6.79	-11.75	539.33
1.74	580.08	-77.04	503.04	32.13	-6.52	-11.31	517.34
1.46	505.94	-46.89	459.05	32.50	-5.98	-10.90	474.67
1.26	452.41	-54.75	397.66	32.70	-5.24	-10.51	414.61
1.05	392.71	-43.77	348.93	32.90	-4.65	-10.14	367.04
0.80	308.64	-25.98	282.66	33.05	-3.84	-9.79	302.08
0.56	223.08	-17.41	205.67	33.26	-2.91	-9.46	226.56
0.26	109.45	-5.06	104.40	33.25	-1.68	-9.15	126.83

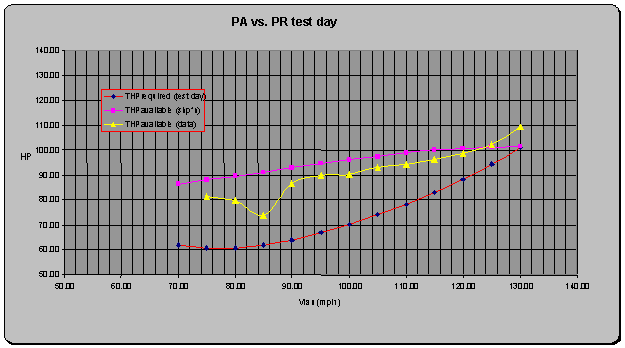
A plot of R/C_{test day} and R/C_std is shown in the figure on the next page.

Figure 9 R/C 3000ft 2200 lbs std day

Test Day: 2175 lbs, OAT 66° F

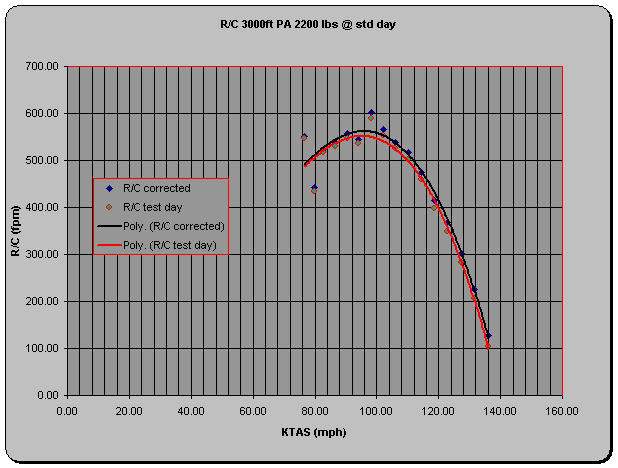
Normally, correcting the results to W_std equal to 2200 lbs would result in a decreased R/C, but test day weight of 2175 lbs is only 25 lbs lighter than W_std of 2200 lbs, whereas, test day temperature was nearly 20° hotter resulting in a predicted increased R/C_std.

The next step is to combine the calculated values of excess power with power required data from the previous flight test. To do this, R/C must be converted to power available according to the following relationship:

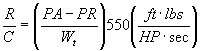, solving for PA (in HP)

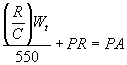Combining the two plots (power available and power required) requires the standardized power required curve to be corrected to the same values of W_std and T_std the R/C of data was corrected for previously, otherwise results will not be valid. An alternate method of calculating power available is accomplished by multiplying the engine shaft horsepower (SHP) by propeller efficiency, η. The result of which is thrust horsepower available. The values of SHP are determined from manifold and rpm settings cross-referenced to the manufactures power chart with corrections for altitude and temperature applied. Propeller efficiencies were generated using Hartzell’s propeller performance mapping software. A plot of power available calculated using both methods and power required curve is shown on the next page.

Figure 10 Power Available vs. Power Required 3000ft

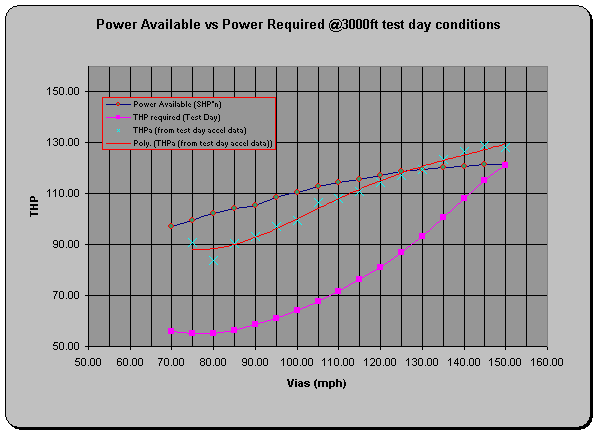
As can be seen from the plot above, values of power available calculated by multiplying shaft horsepower by prop efficiency intersects the power required curve at V_max as expected. Failure of the data calculated from rate of climb to intersect this point is attributed to errors introduced in the data reduction process, specifically when taking the derivative of curve fitted data, unaccounted for errors in instrumentation and possibly weakness of the theory itself. Reversing the above process to determine rate of climb (using the SHP*η curve) to compare with the results measured during the test results in the figure on the next page.

Figure 11 R/C 3000 ft

From several hundred hours of experience in this particular aircraft the rate of climb plot (yellow) derived from the engine and prop data is much more representative of indicated climb rates than those generated from level acceleration runs. Values of R/C_max (horizontal green line), V_y (vertical green line) and V_x(vertical blue line) are determined graphically. Maximum climb angle occurs when the ratio of R/C to horizontal velocity is a maximum. Graphically this corresponds to the intersection of a tangent line running through the origin as depicted by the blue line. The maximum climb angle is computed by:

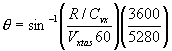. , where V_x is true airspeed in mph.

Similar plots for 1500 and 7500 feet are shown below:

Figure 12 PA vs. PR 1500ft

Figure 13 R/C 1500

Figure 14 PA vs. PR 7500ft

Figure 15 R/C 7500ft

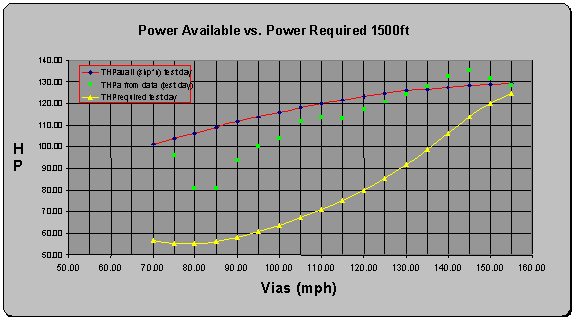
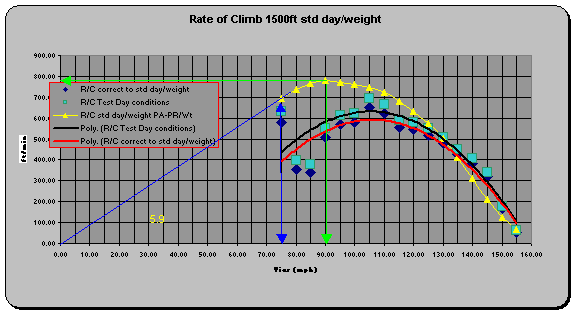
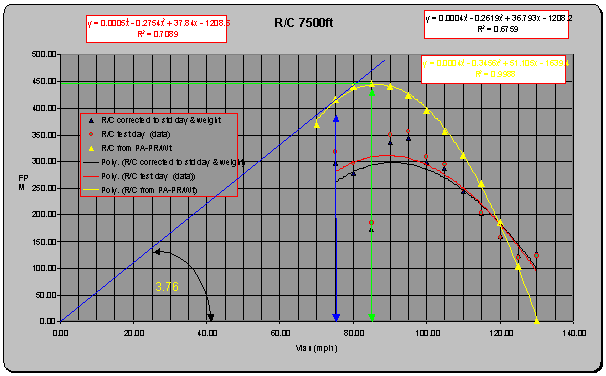
V_y, V_x, R/C_max, AOC_max for all tested altitudes are shown in the table below.

Table 8 Standard Day Climb Performance Values from plots

Altitude (ft)	V_{y (mph)}	V_{x (mph)}	Max R/C (fpm)	Max Climb Angle°
1500	90	75	778	6.05
3000	90	75	740	5.907
7500	85	75	445	3.76

Sawtooth Climb

Similar methodology for data reduction is employed in this method. Static, power, inertial and induced drag corrections are applied as done previously. However, a few added corrections apply only to data measured in this manner. These corrections are:

Tapeline (DR/C_tape) – due to non-standard temp gradient
Acceleration correction (DR/C_accel) – due to change in V_tas during climb.
Wind Gradient Correction (DR/C_wind) – due to wind effects.

Application of static position corrections is complicated in this case by the fact that altitude is changing continuously, therefore requiring the correction be applied at each recorded altitude and for each airspeed as shown in the table below:

Table 9 True Altitudes

gama=	1.4	Ao=	1116.3		True altitude for each airspeed (H_c)
Indicated	Vias	70	75	80	85	90	95	100	110	120
altitude	Vpc	10.09	8.03	6.36	4.75	3.85	2.88	2.03	0.58	-0.59
2500	0.9316	2534.7	2529.6	2525.0	2519.9	2517.1	2513.5	2510.0	2503.2	2496.5
2600	0.928843882	2634.8	2629.7	2625.1	2619.9	2617.1	2613.5	2610.0	2603.2	2596.5
2700	0.926084388	2734.9	2729.8	2725.2	2720.0	2717.2	2713.6	2710.1	2703.2	2696.5
2800	0.923324895	2835.0	2829.9	2825.3	2820.1	2817.2	2813.6	2810.1	2803.2	2796.5
2900	0.920565401	2935.1	2930.0	2925.3	2920.1	2917.3	2913.7	2910.1	2903.2	2896.5
3000	0.917805907	3035.2	3030.1	3025.4	3020.2	3017.3	3013.7	3010.2	3003.2	2996.4
3100	0.915080169	3135.3	3130.1	3125.5	3120.2	3117.4	3113.7	3110.2	3103.2	3096.4
3200	0.91235443	3235.4	3230.2	3225.6	3220.3	3217.4	3213.8	3210.2	3203.2	3196.4
3300	0.909628692	3335.5	3330.3	3325.6	3320.4	3317.5	3313.8	3310.3	3303.2	3296.4
3400	0.906902954	3435.7	3430.4	3425.7	3420.4	3417.5	3413.9	3410.3	3403.2	3396.4
3500	0.904177215	3535.8	3530.5	3525.8	3520.5	3517.6	3513.9	3510.3	3503.3	3496.4
	Sigma std

The uncorrected average R/C for each airspeed is then determined by dividing the difference between measured true altitudes by Dtime.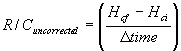

The result of this calculation is shown in the table below.

Table 10 Average Rate of Climb

		Average	Rate of	Climb
Altitude	70	75	80	85	90	95	100	110	120
2600	731.57	762.09	923.76	612.61	1035.01	689.93	1200.36	833.41
2700	747.97	770.90	723.43	1035.10	882.80	645.42	789.71	625.06	299.97
2800	693.56	717.48	1035.26	577.27	968.24	472.63	521.90	882.44	681.75
2900	818.30	481.97	1154.72	698.09	682.17	689.94	480.15	833.41	454.50
3000	721.92	687.12	800.61	833.84	612.56	822.26	638.49	571.48	555.50
3100	650.03	813.74	779.81	750.45	800.41	909.46	857.40	705.95	571.37
3200	638.97	928.19	857.80	870.09	612.56	895.89	1091.24	759.57	799.91
3300	554.09	678.58	594.51	811.30	750.39	769.55	822.17	631.64	705.81
3400	681.00	792.28	706.43	645.56	582.83	845.42	659.54	600.06	491.75
3500	691.19	753.51	594.52	741.20	682.18	857.50	895.80	526.37	472.39
	692.86	738.59	817.09	757.55	760.92	759.80	795.68	696.94	559.22

The bold faced values along the bottom row of the chart are the averaged rate of climbs for each airspeed from 70-120 mph. At this point the values are corrected only for static position errors.

Because altimeters, the primary data source in this method, are calibrated to changes in static pressure at standard sea-level conditions, circumstances in which temperature is non-standard introduces errors in H_i. To correct for these errors the following equation is applied:

The next correction to be applied is the power correction used to compensate for variations in engine output with changes in inlet temperatures. This correction is applied in the same manner as was done during level acceleration runs.

During the climb static ambient pressure decreases as does temperature (normally), therefore air density decreases as altitude increases according to the equation of state:

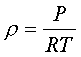
as ρ decreases the value of σ, σ = (ρ/ρ_{sea level}), decreases and since , V_tasincreases with altitude. Therefore while climbing at constant V_ias (or V_cas) , V_tas is increasing with altitude, hence the term flight path acceleration error. The correction for this error is determined by:

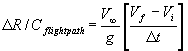The wind gradient was neglected in this test because an accurate method for wind speed determination was not available on test day. An effective and simple method for determining the wind gradient, change in wind speed with altitude, is through use of GPS ground speed calculation. Subtracting V_ground from V_tas results in wind velocity. The slope of a plot of wind velocity for each altitude determines the wind gradient.

The wind gradient correction is given by:

Because the weight of the aircraft is continuously decreasing during the test a weight correction must be applied to maintain validity of the results. Consulting manufactures fuel consumption charts to retrieve consumption rates during the test and using real times recorded during each run allowed the following table of tabulated gross weights to be generated.

Table 11 Averaged Aircraft Gross Weight

Aircraft Weight Data		Ws=	2200	AR=	6.4	e=	0.7031	s=	102.33
Climb burn Rate	13						0.6507
Descent BR	5
IAS (mph)	70	75	80	85	90	95	100	110	120
start GW	2028.91	2025.32	2021.80	2018.37	2014.87	2011.94	2009.00	2006.06	2002.46
End GW	2026.76	2023.27	2019.91	2016.37	2012.86	2009.92	2006.99	2003.89	1999.90
Ave GW	2027.84	2024.30	2020.85	2017.37	2013.87	2010.93	2008.00	2004.98	2001.18

Finally, corrections for non-standard weight (inertial and induced drag) applied to the level acceleration data is also applied here to result in standard day rate of climb corrected for static, power, flight path acceleration, weight, inertial and induced drag effects. A plot of sawtooth generated R/C is shown in the figure below. The complete data table and results can be found in the appendix.

Figure 16 Sawtooth R/C 3000ft

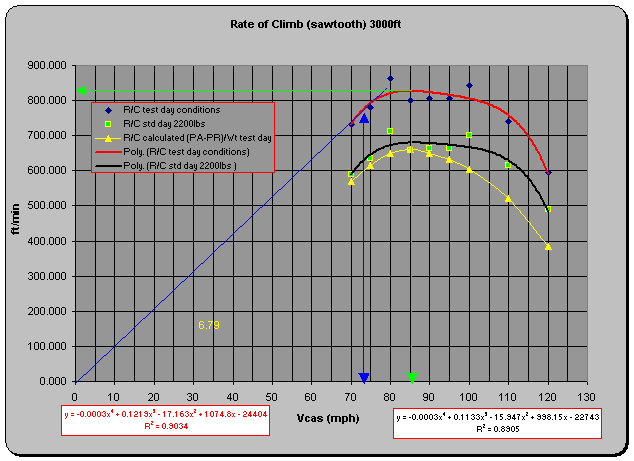
Notice both V_y and V_x agree well with level acceleration data. However, data points are not very smooth by any means. This could be attributed to the neglected wind gradient correction, errors in temperature measurement and quite possibly the airplane itself may exhibit nonlineararities at various points within its envelope (no pilot error noted during data collection!). To check the validity of one method over the other, a combined plot of standard day and weight R/C for both level acceleration and sawtooth climb techniques is shown in the figure on the next page.

Figure 17 Combined R/C Plot

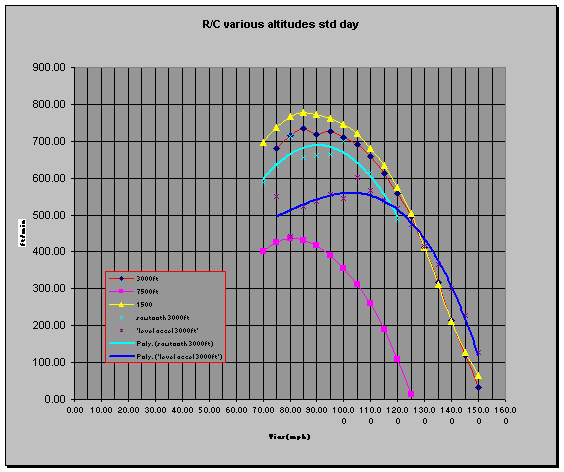
This plot shows nice agreement between the sawtooth and the R/C curve calculated from SHP*η at 3000 feet. The 3000ft level acceleration run however does not match well with the other data, thereby confirming the common understanding this method is not desirable for low performance aircraft.

Having now validated the method of using engine charts and prop software to determine power available, the next step is to find usefulness for the information generated.

Data Application

With the data thus presented the task at hand is to put it into useful form for operational use. Excess power has important effects on aircraft performance and is a particularly important aspect of the preflight planning phase of any flight. Pilots must determine whether or not the aircrafts performance will ensure safe operation in the environment in which they will be operating. Cross-country flying requires detailed planning to increase the likelihood of a successful trip. All to often complacent pilots set out on a journey not having consulted current conditions and aircraft performance specifications as to whether the aircraft can indeed meet the performance required to ensure a safe outcome. Climb gradient, time to climb and fuel to climb are all very important considerations which must be reviewed prior to flying in unfamiliar territory or under adverse weather conditions. The purpose of this section is to transform test data to useful information for safer and more consistent operation.

Before the information can be transformed however, it is first necessary to develop relationships between the data collected over a broader range of altitudes and weights. By tabulating and plotting the values of R/C_max for each altitude tested (1500, 3000, 7500ft) while varying the W_std corrections over a range of gross weights from 1800-2200, the relationship between R/C_max and altitude is generated for fixed gross weight. Next a plot of R/C_max vs. weight is generated for each of the three tested altitudes to generated a relationship between R/C_max and weight for a fixed altitude. Curve fit equations are generated and applied to a range of weights and altitudes thus generating a table of R/C_max for the weights and altitudes of concern. These relationships are then applied to R/C_aocmax to determine the climb angles at various weights and altitude as well.

These plots are shown in the figures below.

Figure 18 R/C max vs. Weight

Figure 19 R/Cmax vs. Altitude

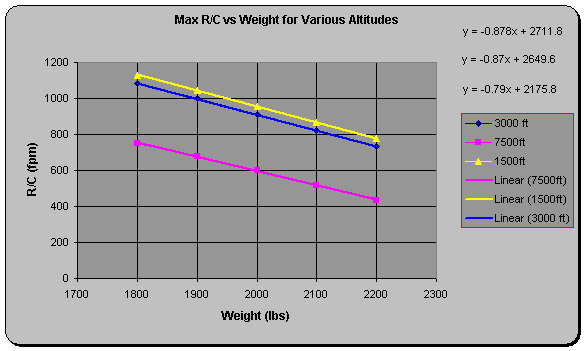
Climb Gradient

Climb gradient is a very important performance parameter to consider especially when conducting Standard Instrument Departure (SIDs) procedures under instrument meteorological conditions (IMC). Often SIDs specify the aircraft be able to maintain a minimum climb gradient until a specific point is reached on the departure to ensure adequate obstacle clearance with ground or man-made objects. For example, a note written in the SECA2 departure from Monterey Peninsula Airport (KMRY) states,

“Note: This SID requires a minimum climb of 405’ per NM to 4000’”. This is due to close proximity of the departure course to Jacks Peak, a 3300 ft mountain. Solving for maximum climb gradient requires the maximum climb angle to be known for each altitude and weight of interest. Maximum climb angle is determined as previously discussed. Once obtained, climb gradient is determined by solving the trigonometry problem depicted below:

Figure 20 Climb Gradient Triangle

R/C_aocmax

Horizontal Distance Traveled (nm)

The tables below show the result of this calculation.

Table 12 Climb Angle and Gradient

Max Climb Angle (deg) std day							Altitude
Weight	0	1000	2000	3000	4000	5000	6000	7000	8000	9000	10000	11000	12000
1800	8.51	8.22	8.10	7.86	7.50	7.02	6.42	5.70	4.86	3.90	2.82	1.62	0.30
1900	7.81	7.52	7.40	7.16	6.80	6.32	5.72	5.00	4.16	3.20	2.12	0.92	-0.40
2000	7.11	6.82	6.70	6.46	6.10	5.62	5.02	4.30	3.46	2.50	1.42	0.22	-1.10
2100	6.41	6.12	6.00	5.76	5.40	4.92	4.32	3.60	2.76	1.80	0.72	-0.48	-1.80
2200	5.71	5.42	5.30	5.06	4.70	4.22	3.62	2.90	2.06	1.10	0.02	-1.18	-2.50

Max Climb Gradient ft/nm std day							Altitude
Weight	0	1000	2000	3000	4000	5000	6000	7000	8000	9000	10000	11000	12000
1800	909	878	865	839	800	748	684	607	517	415	300	172	32
1900	833	802	789	763	725	673	609	532	442	340	225	98	-42
2000	758	727	714	688	650	598	534	457	368	266	151	24	-116
2100	683	652	639	613	575	523	459	383	293	191	77	-50	-190
2200	608	577	564	538	500	449	385	308	219	117	3	-125	-265
Add/subtract 4 ft/nm for every 30 degrees above/below standard atmospheric temperature.

Gradient temperature dependence curve is shown in the figure below.

Figure 21 Gradient Temperature Dependence

Time to Climb

Time to climb information is useful for choosing optimum cruising altitudes for given wind conditions in consideration of the total distance to be traveled. Assuming the climb will be conducted @ V_y and under maximum power, the time to climb can be determined by:

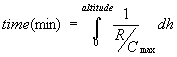
graphically this is determined by calculating the area under the curve of 1/(R/C_max) vs. altitude as shown below:

Figure 22 Time to Climb

Time to Climb

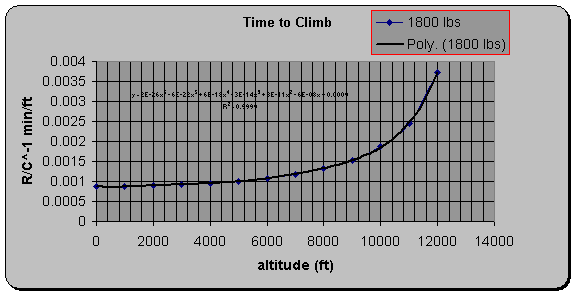
Integrating the six order polyfit curve and applying the altitude and weight relationships determined previously results in the following R/C_max and Time to Climb tables.

Table 13 R/C_max

						Max R/C
						Altitude (ft)
weight (lb)	0	1000	2000	3000	4000	5000	6000	7000	8000	9000	10000	11000	12000
1800	1150	1143	1123	1092	1048	993	925	846	754	651	535	408	268
1900	1063	1055	1036	1004	961	905	838	758	667	563	448	320	181
2000	975	967	948	916	873	817	750	670	579	475	360	232	93
2100	887	880	860	829	785	730	662	583	491	388	272	145	5
2200	799	792	772	741	697	642	574	495	403	300	184	57

Table 14 Time to Climb

					Time to Climb @ Vy full power					minutes
	0	1000	2000	3000	4000	5000	6000	7000	8000	9000	10000	11000	12000
1800	0	0.9	1.8	2.7	3.6	4.6	5.6	6.7	8.0	9.4	11.1	13.2	16.2
1900	0	0.9	1.9	2.9	3.9	5.0	6.1	7.4	8.8	10.4	12.4	15.0	19.0
2000	0	1.0	2.1	3.1	4.3	5.4	6.7	8.1	9.7	11.6	14.0	17.4	23.6
2100	0	1.1	2.3	3.5	4.7	6.0	7.5	9.1	10.9	13.2	16.2	21.0	34.4
2200	0	1.3	2.5	3.9	5.2	6.7	8.4	10.3	12.5	15.3	19.5	27.8

Assuming a full power climb @ V_yand consultation of fuel consumption charts allows calculation of the amount of fuel burned during the climb as shown in the table below.

Table 15 Fuel to Climb

	Alt				Fuel to climb @ Vy max power					13 gal /hr
GW	0	1000	2000	3000	4000	5000	6000	7000	8000	9000	10000	11000	12000
1800	0	0.2	0.4	0.6	0.8	1.0	1.2	1.5	1.7	2.0	2.4	2.9	3.5
1900	0	0.2	0.4	0.6	0.8	1.1	1.3	1.6	1.9	2.3	2.7	3.2	4.1
2000	0	0.2	0.4	0.7	0.9	1.2	1.5	1.8	2.1	2.5	3.0	3.8	5.1
2100	0	0.2	0.5	0.8	1.0	1.3	1.6	2.0	2.4	2.9	3.5	4.6	7.5
2200	0	0.3	0.5	0.8	1.1	1.5	1.8	2.2	2.7	3.3	4.2	6.0

Absolute and Service Ceilings

Determination of absolute and service ceilings is a simple matter of plotting altitude vs. R/C_max and noting the intersection of the resulting curve on the altitude scale as shown in the figure below.

Figure 23 Absolute and Service Ceilings

Service Ceiling (100 fpm)

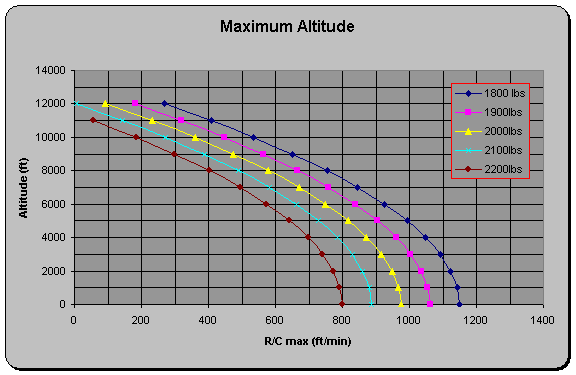
Table 16 Absolute and Service Ceilings

Weight	1800	1900	2000	2100	2200
Service Ceiling (ft)	13,200	12,600	12,000	11,400	10,600
Absolute Ceiling (ft)	14,000	13,500	12,600	12,000	11,400

These values match very well with actual altitude limits experienced during several hundred flying hours in this airplane.

Conclusions

With the data and tables calculated in this report operation of the Kopp BD-4 can be conducted in a more safe and consistent manner. Level acceleration runs are both challenging to fly and limited in their ability to offer accurate data for relatively low performance aircraft such as this (it kills me to say this). Further sawtooth climb testing would enable further validation of the engine power charts and propeller mapping software. Data can now be added to the summary table as shown below.

Table 17 Performance Summary Table

Altitude / Weight	Max C_l/C_d	Min Thrust Required
3000 ft / 1950 lbs	8.8235	217.87 lbs
7500 ft / 2130 lbs	9.0329	229.63 lbs
Parameters	Drag Polar	Power Curve
C_do	0.0440	0.0425
e	0.7031	0.6507
Altitude	Minimum Thrust Horsepower Required
3500 ft 1950 lbs	52.33 HP
7500 ft 2130 lbs	60.59 HP
Standardized	59.16 HP
V_{x (ias)}	75 mph
V_{y (ias)}	90 mph
R/C_max S.L max GW	799 fpm
AOC_max S.L. max GW	5.71°
Service Ceiling @ max GW	10,600 ft
Absolute Ceiling @ max GW	11,400 ft
Kopp BD-4 Summary Table Test conducted 27 July, 2000 Data to be added upon further testing

Flight test three will be conducted for the purpose of neutral point determination for longitudinal static stability determination.

Appendix

Table 18 1500 ft level accel data

	dh/dt	climb/desc	dh/dt	Power	inertial	ind drag	DH/DT	THPa	THPa	THPa	Viw	Piw	THPr	DH/DT	DH/DT
Dv/dt	uncorrected	correction	test day	correction	correction	correction	corrected	*shpn**	*VThrust**	from data	mcas	hp	hp	PA-PR/Wt	Corrected
(fps)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	101.34	101.53		83.47	61.96	56.57	679.72
3.44	778.34	-145.49	632.85	16.67	-23.11	-45.73	580.69	103.74	103.86	96.11	86.53	60.66	55.37	734.34	696.14
3.36	787.66	-389.94	397.72	17.02	-14.76	-43.96	356.02	106.31	106.35	80.89	90.00	60.57	55.28	774.69	738.11
3.28	799.56	-419.61	379.96	17.40	-14.14	-42.29	340.93	109.05	108.53	80.65	93.53	61.57	56.19	802.60	767.73
3.20	810.72	-258.31	552.41	17.74	-20.28	-40.43	509.43	111.61	111.16	93.75	97.81	63.76	58.18	811.21	778.42
3.08	816.67	-204.67	612.00	18.03	-22.41	-38.77	568.84	113.67	113.76	100.15	102.00	66.58	60.75	803.52	772.77
2.97	819.87	-197.58	622.28	18.33	-22.79	-37.19	580.64	115.90	116.04	103.85	106.33	69.92	63.79	791.17	762.46
2.74	790.35	-97.02	693.32	18.64	-25.33	-35.70	650.93	118.13	118.22	111.84	110.75	73.67	67.21	773.13	746.43
2.43	728.02	-64.09	663.93	18.91	-24.29	-34.31	624.24	120.19	120.27	113.73	115.25	77.83	70.99	746.90	722.19
2.26	705.34	-114.24	591.10	19.08	-21.71	-32.99	555.48	121.57	121.86	113.28	119.81	82.47	75.22	703.62	680.91
2.07	670.85	-93.29	577.56	19.28	-21.23	-31.76	543.83	123.12	123.27	117.20	124.45	87.74	80.02	654.34	633.65
1.85	625.06	-76.85	548.21	19.47	-20.20	-30.60	516.88	124.67	124.51	120.80	129.15	93.76	85.51	594.56	575.95
1.63	568.98	-62.81	506.17	19.64	-18.71	-29.51	477.59	126.05	125.21	124.35	133.92	100.63	91.76	520.53	504.08
1.49	541.36	-90.40	450.97	19.70	-16.75	-28.48	425.45	126.75	126.44	127.78	138.78	108.30	98.75	425.03	410.84
1.15	432.68	-27.68	405.00	19.80	-15.11	-27.50	382.19	127.61	127.14	132.31	143.71	116.51	106.24	324.50	312.61
0.96	372.93	-34.77	338.16	19.87	-12.74	-26.57	318.72	128.30	127.64	135.45	148.73	124.69	113.69	221.94	212.30
0.46	186.90	-7.86	179.04	19.95	-7.08	-25.68	166.22	128.99	127.95	131.66	153.83	131.77	120.14	134.47	126.85
0.15	64.23	-3.05	61.17	19.96	-2.89	-24.46	53.78	129.17	128.81	128.43	161.54	136.55	124.49	71.04	65.43

Table 19 7500 ft accel data

	dh/dt	climb/desc	dh/dt	Power	inertial	ind drag	DH/DT	THPa	THPa	THPa	Viw	Piw	THPr	DH/DT	DH/DT
Dv/dt	uncorrected	correction	test day	correction	correction	correction	corrected	*shpn**	*VThrust**	from data	mcas	hp	hp	PA-PR/Wt	Corrected
(fps)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	(fpm)	86.29	86.07		83.72	61.80	61.95	369.50
1.88	471.78	-153.95	317.83	32.25	-10.30	-42.00	297.78	88.21	87.69	81.33	86.79	60.61	60.74	416.94	401.72
1.78	462.59	-167.02	295.58	32.76	-9.66	-40.37	278.31	89.72	89.35	79.88	90.27	60.61	60.74	439.92	426.55
1.72	463.93	-279.57	184.36	33.27	-6.40	-38.84	172.40	91.23	91.20	73.74	93.81	61.68	61.80	446.75	435.34
1.61	453.23	-103.26	349.97	33.88	-11.29	-37.14	335.42	93.00	93.07	86.72	98.10	63.94	64.06	439.42	430.40
1.40	411.64	-55.78	355.86	34.50	-11.48	-35.61	343.27	94.78	94.66	89.96	102.31	66.80	66.92	422.97	416.29
1.27	389.19	-80.78	308.41	35.01	-10.10	-34.15	299.17	96.28	96.17	90.26	106.65	70.18	70.29	394.63	390.26
1.06	339.87	-45.93	293.94	35.43	-9.69	-32.79	286.90	97.52	97.56	93.11	111.08	73.96	74.07	355.97	353.85
0.88	292.37	-44.35	248.03	35.85	-8.35	-31.50	244.03	98.76	98.48	94.33	115.59	78.16	78.27	311.05	311.17
0.71	244.19	-39.83	204.37	36.27	-7.08	-30.30	203.26	99.99	99.57	96.20	120.17	82.86	82.96	258.57	260.95
0.52	186.14	-27.97	158.17	36.44	-5.72	-29.17	159.72	100.56	100.13	98.54	124.82	88.19	88.29	186.23	190.79
0.39	145.87	-26.44	119.43	36.61	-4.59	-28.10	123.35	101.12	100.88	102.12	129.53	94.29	94.39	102.20	109.02
0.35	134.11	-10.61	123.50	36.74	-4.71	-27.10	128.43	101.55	101.07	109.33	134.32	101.24	101.34	3.22	12.37

Table 20 Sawtooth Raw data

Start fuel	Gr Wt	Wind	Altimeter	Temp	Start	T/O	Climb	level	Transit
(gal)	(lbs)	(knots)	(in. Hg)	(deg F)	Time	Time	Power	time	Power
42	2052.6	300/9	30.02	64	17:04	17:12	28MP/2700	17:15	26MP/2700
Data Runs
Start Time	17:25	17:29	17:33	17:37	17:41	17:44	17:48	17:51	17:55
IAS (mph)	70	75	80	85	90	95	100	110	120
Altitude	El Time	El Time	El Time	El Time	El Time	El Time	El Time	El Time	El Time
2500	0	0	0	0	0	0	0	0	0
2600	8.21	7.88	6.5	9.8	5.8	8.7	5	7.2	??
2700	8.03	7.79	8.3	5.8	6.8	9.3	7.6	9.6	20
2800	8.66	8.37	5.8	10.4	6.2	12.7	11.5	6.8	8.8
2900	7.34	12.46	5.2	8.6	8.8	8.7	12.5	7.2	13.2
3000	8.32	8.74	7.5	7.2	9.8	7.3	9.4	10.5	10.8
3100	9.24	7.38	7.7	8	7.5	6.6	7	8.5	10.5
3200	9.4	6.47	7	6.9	9.8	6.7	5.5	7.9	7.5
3300	10.84	8.85	10.1	7.4	8	7.8	7.3	9.5	8.5
3400	8.82	7.58	8.5	9.3	10.3	7.1	9.1	10	12.2
3500	8.69	7.97	10.1	8.1	8.8	7	6.7	11.4	12.7

Tot El. Time	87.55	83.49	76.7	81.5	81.8	81.9	81.6	88.6	104.2
(sec)

Table 21 Sawtooth reduced data

						Test day	Test day			std	Test day
Vktas	Altitude	delta Ti	SHP	rpm	n	Thrust	THPa	Viw	Piw	THPr	THPr
74.3	3035.23	24.67	158.78	2700.00	0.61	425.00	96.85	84.94	61.17	54.73	61.85
77.0	3030.06	24.67	158.81	2700.00	0.62	419.00	99.09	88.05	60.46	54.10	61.30
80.1	3025.41	24.67	158.83	2700.00	0.64	412.00	101.65	91.59	60.91	54.50	61.90
83.2	3020.18	24.67	158.86	2700.00	0.65	405.00	103.90	95.18	62.31	55.76	63.50
87.0	3017.33	24.67	158.88	2700.00	0.67	397.00	105.97	99.53	64.86	58.03	66.26
90.8	3013.70	24.67	158.90	2700.00	0.68	388.00	108.05	103.80	67.92	60.78	69.55
94.6	3010.17	24.67	158.92	2700.00	0.69	380.00	110.13	108.20	71.47	63.95	73.34
102.6	3003.20	24.67	158.96	2700.00	0.72	362.00	113.81	117.28	79.83	71.44	82.11
110.8	2996.44	24.67	158.99	2700.00	0.73	343.00	116.70	126.64	90.46	80.94	93.30

				Flight Path Acceleration Correction			measured	Tapeline Alt	Power		Test Day	Inertial	Ind drag	Std day 2200lbs	calculated
Ias	Vpc	Cas	Vtas initial	Vtas target	Vtas final	Dv/dt	Dh/dt	correction	correction	Accel corr	DH/DT	correction	correct	DH/DT	DH/DT
mph	mph	mph	mph	mph	mph	fps	fpm	fpm	fpm	fpm	fpm	fpm	fpm	fpm	fpm
70	10.09	80.09	84.87	85.49	86.20	0.0224	692.86	726.57	37.00	5.200	731.774	-65.27	-114.21	589.296	569.62
75	8.03	83.03	87.98	88.62	89.36	0.0244	738.59	774.53	37.92	5.860	780.385	-71.03	-112.54	634.741	616.14
80	6.36	86.36	91.51	92.18	92.95	0.0276	817.09	856.84	38.97	6.901	863.744	-80.02	-110.42	712.271	649.07
85	4.75	89.75	95.10	95.80	96.60	0.0270	757.55	794.41	39.90	7.014	801.426	-76.16	-108.41	656.747	660.84
90	3.85	93.85	99.44	100.17	101.01	0.0281	760.92	797.94	40.77	7.642	805.583	-78.22	-105.76	662.367	650.67
95	2.88	97.88	103.71	104.47	105.34	0.0293	759.80	796.77	41.63	8.301	805.072	-79.61	-103.09	664.004	631.84
100	2.03	102.03	108.10	108.90	109.81	0.0306	795.68	834.39	42.49	9.053	843.445	-84.71	-100.51	700.715	604.63
110	0.58	110.58	117.17	118.03	119.02	0.0306	696.94	730.85	43.98	9.795	740.646	-76.32	-94.26	614.041	521.84
120	-0.59	119.41	126.53	127.45	128.52	0.0281	559.22	586.43	45.18	9.711	596.137	-63.72	-89.08	488.519	385.90

Altitude / Weight

Parameters

Drag Polar

Power Curve

Cdo

0.0440

0.0425

e

0.7031

0.6507

Wing Span